Philosophy of Math: What math would aliens know? (Clement Hongler) | Ep. 12

Опубликовано: 03 Февраль 2021
на канале: Daniel Rubin

1,614

Clement Hongler is a professor of mathematics at EPFL in Lausanne, Switzerland. We discuss some issues in the philosophy of mathematics, namely the relationship of mathematics to the real world, how math can be "natural" or historically contingent, the prospects of fundamental or unified theories of math or the real world, and what the mathematics of intelligent alien life might look like.

Previous episode with Clement: • Mathematics of Machine Learning and t...

Links:
Type theory: https://en.wikipedia.org/wiki/Type_th...
Plimpton 322 tablet: https://mathcs.clarku.edu/~djoyce/mat..., https://blogs.scientificamerican.com/...
Learning prairie dog language: https://blogs.scientificamerican.com/...
How the brain determines spatial position: https://www.biorxiv.org/content/10.11..., https://fietelabmit.files.wordpress.c...
Platonism: https://plato.stanford.edu/entries/pl...
Wigner's essay: https://onlinelibrary.wiley.com/doi/a...
Weil conjectures: https://en.wikipedia.org/wiki/Weil_co...
Solomonoff-Kolmogorov-Chaitin complexity: https://en.wikipedia.org/wiki/Kolmogo...
T.S. Kuhn's "The Structure of Scientific Revolutions": https://en.wikipedia.org/wiki/The_Str... https://amzn.to/3rlDVhB
(I get a small commission from purchases made from this link.)

0:00 What math would aliens know? Imagining a different track of human development
8:01 Pure vs. applied math
11:42 The key issues: historical contingency, factors affecting progress, connection to real experience.
16:50 Why did modern cryptography appear so late?
18:45 Naturalness in math. Getting perspective from experts in other fields
23:57 Naturalness as a matter of fitting into a general framework. Kolmogorov's axioms of probability. Set theory vs. type theory. Contingency of chosen definitions.
32:00 Numbers, finite symmetry groups, and combinatorics
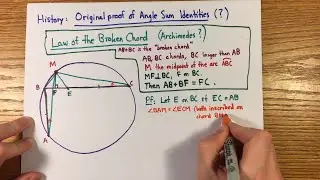
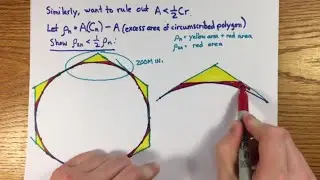
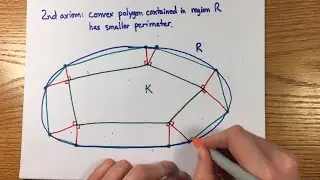
36:15 Examples that offer a glimpse at mathematical systems different from ours: Ex. 1, Babylonian mathematics.
44:03 Ex. 2, the movie Arrival and deciphering animal languages
51:40 Ex. 3, how the brain computes and communicates. Neuralink, grid cells and position cells.
59:10 What is math? Abstractions and theorem-proving. Relation of math to the real world and other disciplines.
1:07:59 Could we have developed calculus without experience of the real world? Could the results of calculus be reproduced with only discrete mathematics?
1:12:42 Role of real-world experience and intuition in math. Can computers outperform humans at completely abstract, disconnected mathematics?
1:18:44 Platonism and the discovery/invention debates. Wigner's essay "The unreasonable effectiveness of mathematics in the natural sciences." Patterns and selection bias.
1:32:40 Why is math less effective in biology, history, the arts?
1:44:48 Fundamental/unified theories of physics/math. Tests of explanatory power and efficiency of explanation.
1:53:29 What is the value of unification?
1:57:59 Unified theories of math. Axioms, Grothendieck's attempt to find the "true" mathematics. The Weil conjectures.
2:02:00 Does the universe have a "source code"? Solomonoff complexity
2:06:00 The need for experiment and crisis for progress in theory. Could quantum mechanics have been anticipated without the experiments of the early 20th century?
2:14:08 The examples of Dirac and Einstein leading people astray. The appeal of string theory.
2:21:53 T.S. Kuhn's "The Structure of Scientific Revolutions." Procedural and epistemological obstructions to finding fundamental theories. Short- and long-term thinking.